Year 3 to Year 6: Three Squares
What is the greatest number of squares you can make by overlapping three squares of the same size?

What is the greatest number of squares you can make by overlapping three squares of the same size?
We’d love to see pictures showing how you worked this out. They do not have to be arranged like the picture above that is just a possibility. Do you have another way which would result in a greater number of squares? We would also like to know how you go about the task. How will you know if your way really does have the greatest possible number of squares?
Good luck!
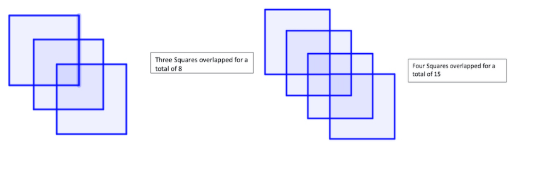
In this problem, we had to make the most squares as it is possible. I made eight squares using three squares and using four squares I made 13 squares.
You play this game like you have to overlap the squares and make many squares. My method was to keep trying different shapes with squares.
I got eight squares by putting two square edge to edge and put the last one in the middle.
I got 13 squares by same as how I got eight squares but I put one more in the bottom.